Sam H. Jones
The logarithmic function, in base 10, f(x) = Log(x), will be utilized in measuring sound levels in decibels. We humans are equipped with sensitive ears capable of detecting sound waves of quite low intensity. The difference in magnitude between what we call the auditory threshold, the softest noise audible to a normal ear, and the loudest audible noise is huge. For example, the magnitude of difference between the auditory threshold and the threshold of pain is some 10,000,000,000,000,000 (ie., ten to the thirteenth power). Logarithms, like scientific notation, are useful when trying to represent or communicate such large magnitudes.
Since the decibel (dB) scale is one of proportion between the baseline, auditory threshold, and an observed or recorded phenomenon the actual unit of measure is not important as the ratio will remain the same. The ratio can be expressed in the following equation: dB = 10*log
10
(P
1
/ P
0
) where is the baseline, or auditory threshold, and is the observed or recorded sound level. While the magnitude of difference between, say a whisper, and normal conversation may appear to be a magnitude of two or three it is actually much larger. An engaging exercise for students might be having them guess the magnitude of difference of some common sounds and then compare with what the actual dB level is.
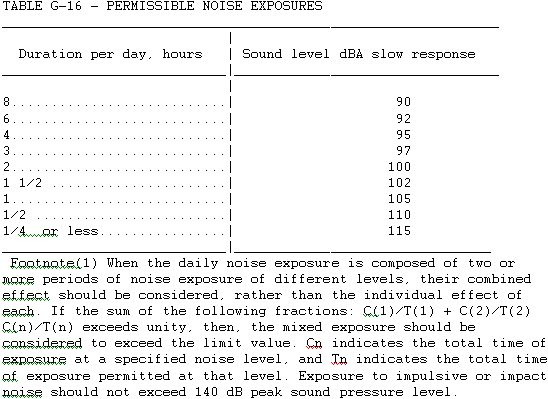
Below are OSHA permissible noise levels. For example, a normal conversation or TV would be 60 dB, a jack hammer about 100 dB, a major highway also about 100 dB, and a jet taking off 150 dB (http://en.wikipedia.org/wiki/Sound_pressure).
Another application of logarithms is calculating gestation periods. For example, if we know that a cell will divide approximately each week creating two cells then we can calculate how long it will take for an embryo to fully develop.
As an example we can use the development of the human brain. The newborn has about one hundred billion neurons. The formula to represent this growth pattern is N = 2
x
. If N equals one hundred billion we can then solve, using the logarithm base 2 as follows: x = log
2
100,000,000,000. Using a calculator we find that x is approximately 36.54 weeks.
Other examples may also be used. The graph of log
10
(x) is shown in figure 3.
Figure 3. source: Wikimedia