The ideal gas law is an equation of state for a gas, where the state of that gas is its condition at a given time. A particular state of a gas is described by its pressure (P), volume (V), temperature (T) and number of moles (n). It is important to recognize that the ideal gas law is an empirical equation - it is based on experimental measurements of the properties of gases. A gas that obeys this equation is said to behave ideally. In other words, an ideal gas is defined as a gas for which PV/(nT) is constant for all pressures, volumes and temperatures. Therefore, the ideal gas is a hypothetical substance. However, most gases obey the ideal gas law closely enough at pressures below one atmosphere (1 atm), that only minimal errors result from assuming the ideal gas behavior.
The number of moles of a gas (
n
) inside a container, is closely related to its temperature, pressure, and volume. This is summarized by the
ideal gas law
which itself is derived from the combined gas law (
Clapeyron-Mendeleev Law
).
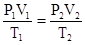
The ideal gas law is given by the equation PV = nRT.
The proportionality constant or gas constant, R, is approximately 0.082 (liter x atmosphere)
/
(Kelvin x mole), or 8.31 Joule/ (mole x Kelvin), or 1.987 calories / (mole x Kelvin). If higher densities of gases are being studied, then corrections to this equation must be made, since they will not behave ideally.